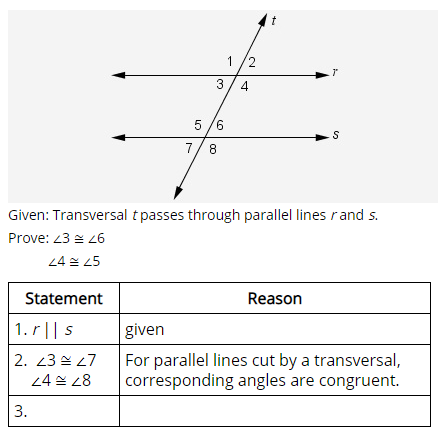
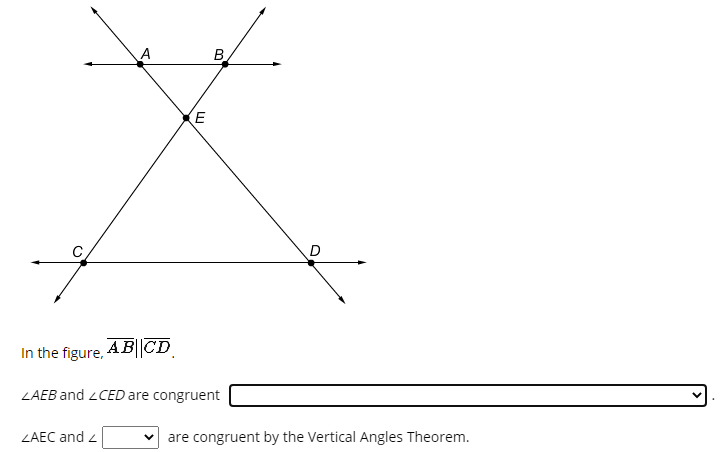
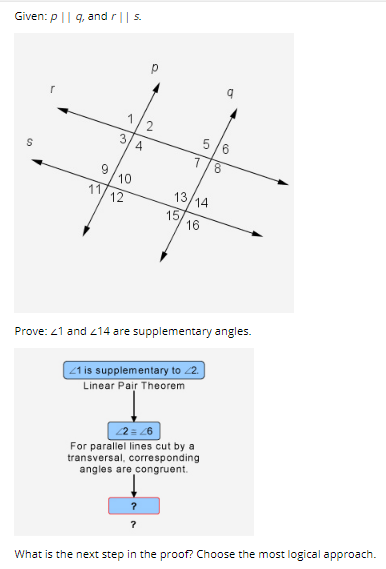
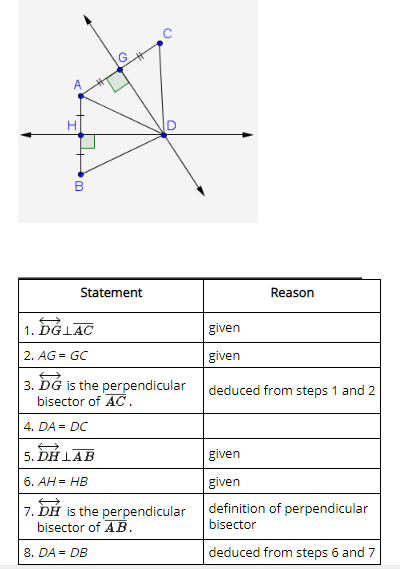
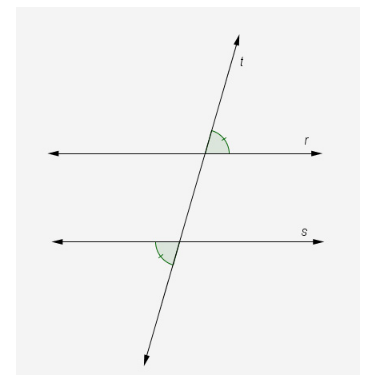
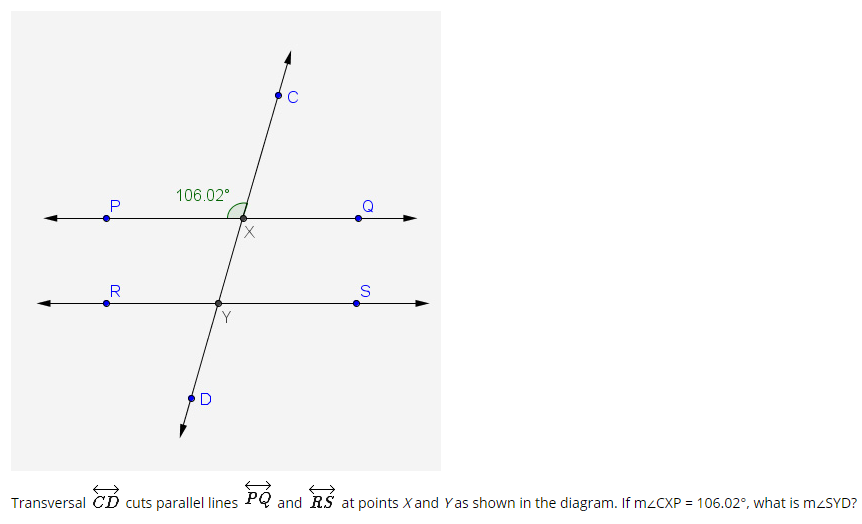
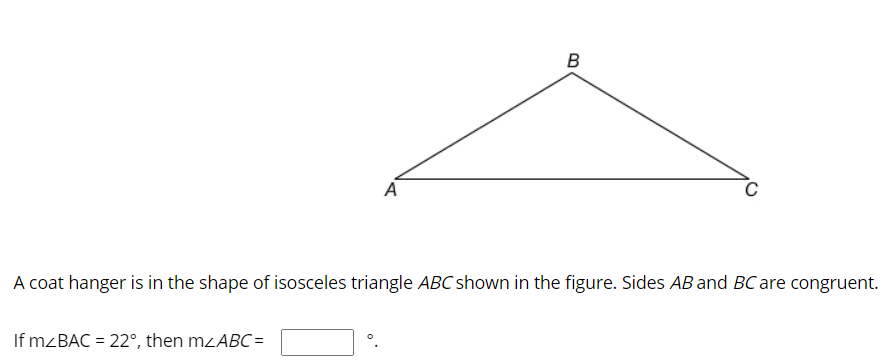
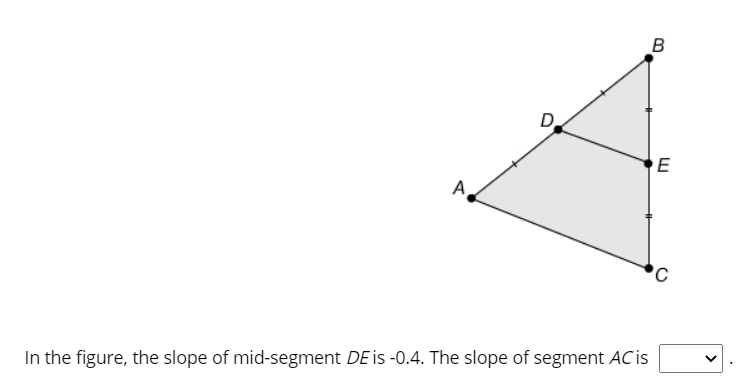
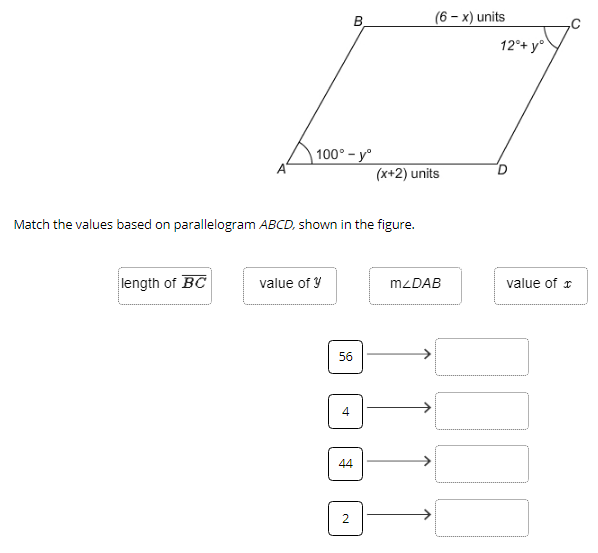
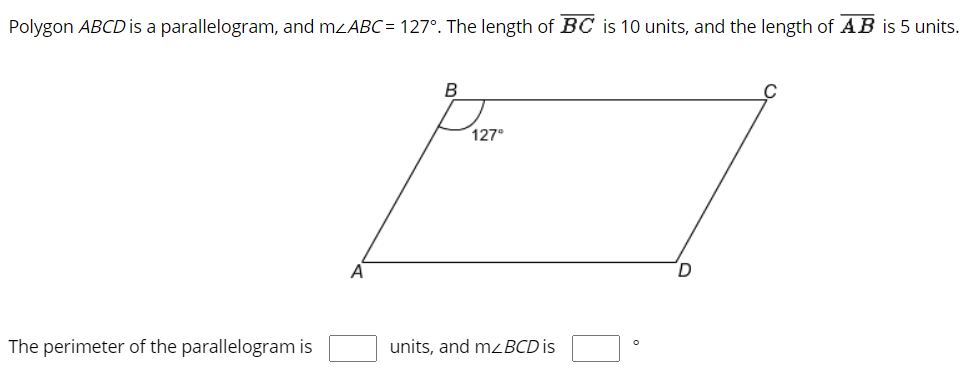
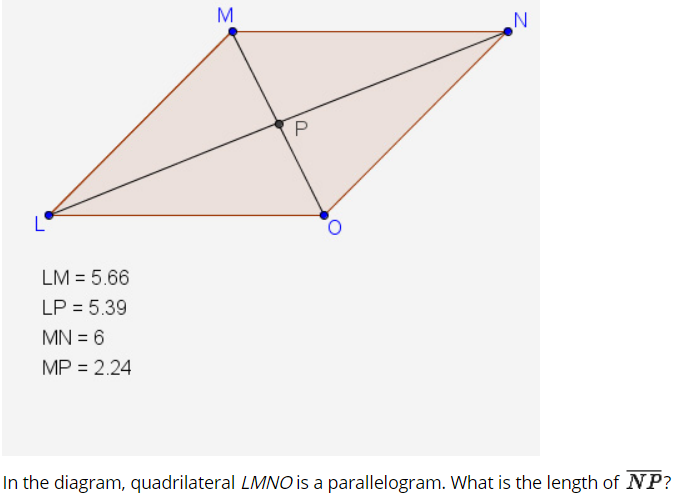
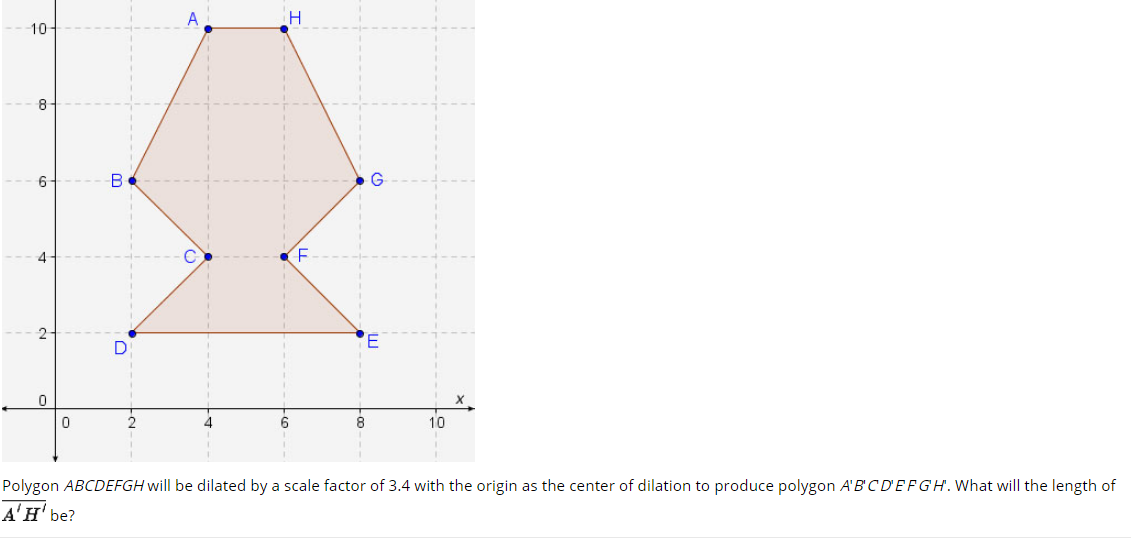
Lines, Angles, and Mathematical Proofs, Proving Theorems about Lines and Angles, Proving Theorems about Triangles, Proving Theorems about Parallelograms, Properties of Dilations, Similarity and Similarity Transformations, Similarity, Proportion, and Triangle Proofs, Using Congruence and Similarity with Triangles,
Angeheftet an
422
0
0
Keine Merkmale angegeben
|
|
Erstellt von Elexali Olayvar
vor etwa 4 Jahre
|
|
Schließen