Required formulae for the new maths GCSE - these will NOT be given during the exam, they must be learned in advance
Pinned to
25
4
0
No tags specified
Close

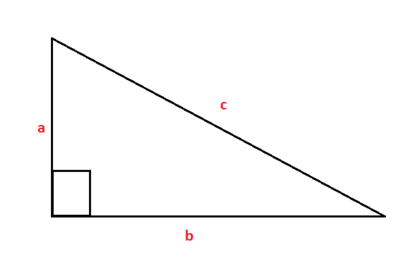
Pythagoras' theorem:
Right-angled triangle: a, b and c are the length of the sides and c is the hypotenuse:
Quadratic Formula - solve:
\(a\)\(x^2\)+\(b\)\(x\)+\(c\)=\(0\)
where \(a\) \(\neq\) \(0\)
Circumference of a Circle:
Area of a Circle:
Trig: In any right-angled triangle \(ABC\) where \(a\), \(b\) and \(c\) are the length of the sides and \(c\) is the hypotenuse:
\(sinA\)=
Trig: In any right-angled triangle \(ABC\) where \(a\), \(b\) and \(c\) are the length of the sides and \(c\) is the hypotenuse:
\(cosA\)=
Trig: In any right-angled triangle \(ABC\) where \(a\), \(b\) and \(c\) are the length of the sides and \(c\) is the hypotenuse:
\(tanA\)=
Sine Rule:
Cosine Rule:
Trigonometry: Area of a Triangle
Area of a Trapezium=
(Where \(a\) and \(b\) are the lengths of the parallel sides and \(h\) is their perpendicular separation)
Volume of a Prism:
Compound interest: Where \(P\) is the principal amount, \(r\) is the interest rate over a given period and \(n\) is number of times that the interest is compounded,
Total accrued=
Where P(A) is the probability of outcome A and P(B) is the probability of outcome B:
P(A or B) =
Where P(A) is the probability of outcome A and P(B) is the probability of outcome B:
P(A and B)
Curved surface area of a cone:
Surface area of a Sphere:
Volume of a Sphere:
Volume of a Cone:
Final Velocity \(v\):
Displacement \(s\):
Velocity \(v\)\(^2\):

 Hide known cards
Hide known cards